“Keep exploring. Keep dreaming. Keep asking why. Don’t settle for what
you already know. Never stop believing in the power of your ideas, your imagination,
your hard work to change the world.
------- Barack Obama.
The general form
of QE is ax^2+bx+c =0, where a≠0, a, b, c €R. The equation is of second degree with
variable x. The equation has two roots, may be distinct, equal, complex, depend
on the discriminant of equation. (D= b^2 -4ac) There are conditions for the
kinds of roots. In this article let me write on applications QE.
Sridhar Acharya , an Indian mathematician born in 8th Century in India. He was a Sanskrit scholar and a mathematician. His father Baldev Acharya was also a Sanskrit Scholar. Sridhar Acharya was the first mathematician who derived the formula of quadratic equation. The above formula is due to Sridhar Acharya. Sridhar Acharya wrote - Pati Ganit, Trisatika and Pati ganit Sara.
1.
A
river flows at 3kmper hour A boat goes 10km upriver and back again. A trip
takes 2 hours to go and return. Find the speed of the boat.?
We can use the formula Time =Distance / Speed. Let us assume the speed of the boat be X Km per hour. As per given data we can form statement: Speed of the boat against the current of water is (X-3) km/hr, in the direction of flow of water is (X+3) Km per hour.
Hence
(10 / X-3) + (10/ X+3) = 2 hours total time taken.
By solving we get the
equation 2X^2 -20X -18= 0,
i.e. X^2 – 10X-9 = 0, we will get X = 10.83Km per
hour. Speed of the boat is 10.83km /hour (up to two decimal places)
2. 2. A
ball is thrown straight up from 9 m above the ground with velocity of 12m/sec. When does hit the ground? (Air resistance
being ignored).
Let ‘t’ denote time, h denotes
height, g denotes gravitational force.
To use the formula S = ut + ½ gt^2.
G = 9.8mper Sec ^2, initial velocity is given as 12m/sec. ½ g be approximated
to be 5. instead of 4.9. ultimately the required statement is h= 9 + 12t-5t^2.
Rewriting the equation as 5t^2 -12t-9=0, By solving we get t = 3, other
value of t is negative, not possible. Hence the answer is 3.
We can draw the graph and verify. The graph shall be parabola.
3.
From the given diagram find the area of A and
B. The total area is given as 42Sq cm.
We know Area of Rectangle =
Length x Breath
Given A + B = 42Sqcm, where area A= 4 × x,
Area B=(3x+1) × x
A =4x, B
= 3x^2+x, we get, A+B= 4x+3x^2+x=3x^2+5x;
given total area as 42, so 3x^2+5x=42,
si.e.,
3x^2+5x-42 = 0, by
solving we get x= 3, the other value is neglected being negative. The
factors are (x-3) and 3x+14). Hence A= 12Sq cm, and B= 30Sqcm.
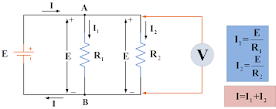
4.In a circuit
diagram two resistors are connected in parallel. The total resistance is
measures as 3ohms.One of the resistors is given to be 8 ohms more than the
other, what are the values of two resistors.?
Since connected in parallel, we can use the
formula 1 / R = (1/ R1) +( 1 / R2) where R denotes total resistance,
R1 and R2 be two resistors. As per given data since one
resistor more than the other by 8, R2 = R1 + 8.
Forming the equation,
we get 1 / 3 = (1 /R1) + (1/ (R1 +8)
3(R1
+ R1 + 8) = R1 (R1 + 8), 6 R1 + 24
= R1^2 + 8 R1, after simplification we get an equation as
R1 ^2 + 2R1 -24 = 0, factors are (R1 +6) and (R1 - -4), hence R1 = 4, the other value is neglected. two resistors’ values are 4 ohms and 12 ohms.
5. If SinA, CosA are the roots of equation 12X^2 +5X-7 =0, Find what is Sin 2A
Sum of the roots SinA + cosA = --5 / 12, product of roots SinA Cos A =
--7 / 12.
Sin 2 A = 2sin A CosA = 2 ×-- 7 / 12 = -- 14 / 12.
(just a linkage with trigonometry, we shall see some more in the next
posting, to be continued)








2 comments:
Sir, thank you very much for your valuable and meaningful article. This article is very exellent and awesome. I am your regular visitor and I always like and share your articles to my friends and your website is awesome.
Kishan
Post a Comment