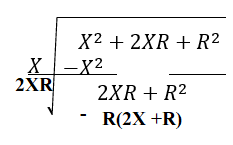April 7, 2023
INDIAN KNOWLEDGE SYSTEM -LECTURE AT G D GOENKA PUBLIC SCHOOL SILIGURI
 An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
March 28, 2023
Women Mathematicians from India ---- K Bhanumoorthy
CONTRIBUTION BY WOMEN IN MATHEMATICS
A quote
from Marie Curie, Noble prize winner & Radio Activity researcher
“We must
have perseverance and above all confidence in ourselves. We must believe that
we are gifted for something and that this thing ,at whatever cost ,must be attained.”
Introduction:
I was given an opportunity to write an article on “The
Importance of Women Education in the Society”, got published on the title of
the book “Women Empowerment and Educational development”. Very recently
people celebrated International Women’s Day on March 8th. Some
organisations recognised the immense services rendered by women on various
fields, had been felicitated with awards and rewards. Since then, I have been
thinking to write an article on the topic given above. There is an assumption
that girls can’t do maths. How can one accept this statement? The assumption is
wrong, if I am permitted to say. Here are the five women Mathematicians from
our country:
1.
Ms. Shakuntala Devi
2.
Ms. Raman Parimala
3.
Dr. Mangala Narlikar
4.
Ms. Sujatha Ramdorai
5.
Dr. Neena Gupta
There are past and present exceptional women mathematicians,
let me share what they have contributed.
1. Shakuntala Devi: She was born on November 4th,
1929 in Bangalore, Karnataka. She redefined women can rule the domain of maths.
She is called as Human Computer. She extracted 23rd root of two
hundred- and one-digit number in 50 seconds. This has been published and listed
in Guinness book of world records in 1980. She has been very popular on mental
calculations. She has also written books, to name few: Puzzles to Puzzle You,
Super memory, etc. She has also touched little bit of the theory of astrology.
4.
Ms. Sujatha Ramdorai: She was born in the year
1962 in Bangalore. She is the first and the only Indian to win ICTP (International
Centre for Theoretical Physics) Ramanujan Prize in 2006, and also Shanti
Swaroop Bhatnagar Award in 2004. She is working in TIFR as a professor in
Maths. She is also member of the scientific committee of several international
research centres; Indo-French centre for Promotion of Advance Research. She is
specialised in pure and applied maths. She worked in the field of arithmetic
geometry of elliptic curves. She said Scientific career helps women to carry
out family life with flexibility, and also said one can achieve excellence by
just understanding Maths.
5.
Dr. Neena Gupta: She was born in the year 1984
in Kolkata. She is an associate professor at the theoretical statistics and
mathematics unit of Indian Statistical Institute (ISI, Kolkata). She is the
youngest to receive Shanti Swaroop Bhatnagar Award in 2019. She has been
recognised for her work in Affine Algebraic Geometry and for suggesting a
solution for the ZARISKI cancellation problem which mathematicians from 20th
and 21st centuries have been trying to solve. She got published her
first research paper on the mathematics puzzle ZARISKI cancellation during the
year 2014. She received the award from Indian National Science Academy also.
Her field of research is mainly on commutative algebra and affine.
I feel happy that I could be aware of eminent people who
have contributed in mathematics. No doubt, many more are there though I have
listed only five in numbers. Let me continue who have contributed from the
other countries in the field of mathematics.
---- be continued
Compiled by :- Mr K Bhanumoorthy (Rtd Principal, KVS)
 An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
February 17, 2023
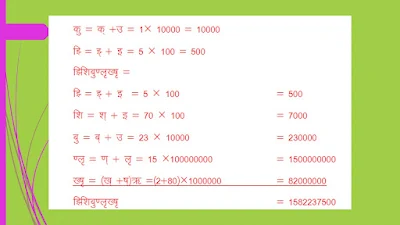
How 7)635( works?
Division is a continued subtraction process.
12 / 4 is basically getting the remainder 0 or less than 3 after repeated subtraction.
12 - 4 = 8 (1st step)
8 - 4 = 4 (2nd step)
4 - 4 = 0 (3rd step)
In order to get the result quickly, we multiply the divisor with a number that gets us closer to dividend. Ex :- 7)635(
Since subtracting 7 repeatedly from 635 will take long time so we reduce this process of subtracting a number by choosing a number by which 7 should be multiplied to get a number closer to 635.
Now look at the question.
In the second step, after subtraction when a digit (5) carried down is less than the divisor (7), we need to place a zero in the quotient. An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
How algorithm to find the Square Root of a number work?
Question:- How algorithm to find the square root of a number works?
Answer:- Square of a number is multiplying a number by itself. Finding the square root of a number is to obtain one factor out of two.
5 x 5 = 25 is called squiring a number.
Square root of 25 is obtained by finding a pair of prime factor and selecting one.
The square root of a number, N, is the number, M, so that M2 = N. The square root algorithm is set up so that we take the square root of a number in the form of (X + R)2. The square root of this number is obviously (X + R). X represents the current approximation for the square root, and R represents the remainder of the number left over from the approximation. Our approximation will always be the correct square root of the number truncated (not rounded) to the number of digits in our approximation. If we expand our number (X + R)2 it will equal X2 + 2RX + R2. This gives us the basis for our derivation of the square root algorithm.
Step 1: The square root of a number between 1 and 100 is a number between 1 and 10.
Step 2: Grouping of a number is done from right to left in pair. The number of groups determine the number of digits a square root of number have.Step 3: Suppose a perfect square root is in the form of X2 + 2RX + R2.
We subtract off the current approximation, X2, which gives 2RX + R2
Step 4: 2RX + R2 = R(2X + R).
Here our current approximation, X, is doubled resulting in 2X, which are the first digits of the number we will be working with.
Step 5: The correct approximation of R will determine the two digits of the square root. This number (R) must divide into the next grouping with the smallest remainder.
Step 6: The procedure can be repeated as many times as necessary until either no remainder is found.
 An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
An author with 60 books, 62 e- books; columnist with 700 articles published in various newspapers and magazines, blogger with 500 + blogs.
India Book of Record Holder for writing 106 books on Mathematics.
My books are published by Rupa, Britannica, Pustak Mahal, Scholastic, Prabhat, Ocean books, Rising Publishers, Amar Ujala, Full Marks etc.
All my books are available on Amazon, Flipkart, Bookadda ,Google books.
My weekly articles get published on Every Wednesday in Amar Ujala Newspaper. I also write column for Dainik Jagaran, Navbharat Times, Vigyan Pragati, Teacher Plus, Aviskar, Vigyan Aapke liye . Vaigyanik Drishtikon etc.
Presently working at DIET Daryaganj as an Assistant Professor.
I am also the Resource person /evaluator working in collaboration with CBSE, NCERT, NIOS, VVM, SSUN, AIRMC, VIPNET, IISF, DST, National Children Science Congress, Sarayu Trust, RIT Chennai, etc.
-
TEST LINK FOR JUNIOR CLASS 8 Test Link for Junior (7) Test Link for Junior (6) TEST LINK FOR CLASS 9 TEST LINK FOR CLASS 10 Test Link for Se...
-
Dear Students SRINIVASA RAMANUJAN, an inspiration for million was born on 22nd December 1887 in Madras Province of British India. His birthd...
-
Dear All On the occasion of Pi Approximation Day being celebrated on 22nd July (22/7) every year, Here is one very interesting quiz dedicat...